Magnetic Field Lines Don't Really Exist

When you equally distribute iron filings over paper and place a magnet underneath, distinct lines form where the filings are more dense alternating with bands of far less density. If you fix sea level, it doesn't matter where on Earth you place an object, the gravitational field strength is the same, 9.8 N/kg. And electric fields are also smooth and uniform (except for the edges). But at least in the middle of the field, the attractive force on a test charge is the same, it doesn't vary. What this makes me think is that magnetic fields are "clumpy" while gravitational and electric fields are not - they are both smooth and uniform. Now if all those assumptions are correct, how could it be that magnetic field strength is the only field whose strength varies so discretely?
- Christopher Swift (age 64)
Thailand

Hi Christopher,

Great question, and one that can easily lead to confusion, due to an accident of nature.

First of all, electric, gravitational, AND magentic fields are all completely smooth. The "field lines" taught in many classes and used by physicists to visualize field strengths are purely to guide the eye; they don't have physical meaning. (Even so, they are useful lines to draw, and even contain weakly quantitative behavior, since the field strength is proportional to the density of field lines. Just remember that between any two such field lines, the field strength is just as strong as on the lines themselves. The field is smooth.)

Ironically, when you do the classic magnet and iron filing experiment, this is what you see:

It sure looks like field lines, right? Actually, this clumpiness has nothing to do with field lines; it's just a coincidence that it looks like lines (or perhaps it inspired the idea of field lines?). The reason for the creation of these pretty iron filing shapes is that each little iron filing, when subject to a magnetic field, becomes a little dipole itself. This dipole feels the force of the magnet, and aligns in the direction of the field lines. In addition, each little dipole feels a small force from the other nearby dipoles, and they move to minimize their local energy. This causes the clumping into lines that you see, as the opposite ends of the dipoles move together.

The clumping is NOT a property of the magnetic field from the large magnet, it is a consequence of the magnetic fields of the small iron filings.

You can do a similar experiment with electric fields instead, and you would expect the same result, since the "test particles" would form electric dipoles and clump. Sure enough, this has been done with grass seeds floating in oil. An electric field from two oppositely charged probes polarizes the seeds into little dipoles, which then align with the field:

In the case of gravity, you can't form such a pattern, because there are no "negative charges" for gravity; objects only have positive mass. In principle, however, if you put together masses, you would get field lines that looked like this:

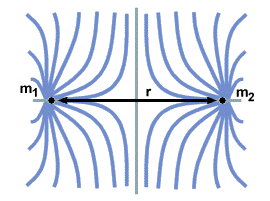
If you then somehow suspended a bunch of point particles in the space between them, they would not align with the field lines, because you can't create mass dipoles (again, there are no negative masses). However, you could use a lot of elliptically shaped masses, which would then rotate so their long axis pointed along the field lines. These patterns would NOT bunch together to form "lines" similar to the first two pictures, because there would be no local dipole interactions. There would be, however, random clumping of particles together due to the local gravitational attractions.

It's hard to do such a visualization, as was done for the magnetic and electric fields above, simply because gravity is too weak on a human scale. The closest example I can think to this is Saturn's rings: You have a bunch of matter particles orbiting peacefully and somewhat smoothly around a planet, but the local interactions cause clumping, which is evident by the substructure of the rings, for example tiny moons, or moonlets (). Such clumping doesn't come from a discrete variation in Saturn's gravitational field, but instead from local interactions of the "test particles."

Hope that makes sense,

David Schmid

p.s. Plenty of other people repeat similar claims which are at best extremely misleading, if not downright wrong. For example, this website () implies that spikes in ferrofluids form around the field lines... no way. The spikes point along the field lines, but they presumably form to minimize the surface tension and gravitational and magnetic potential energy in the experiment. Their discreteness has nothing to do with our imaginary but useful "field lines."

(published on 06/28/2014)